Tag Archives: Chaos
Hopf Fibration and Chaotic Attractors, etc.
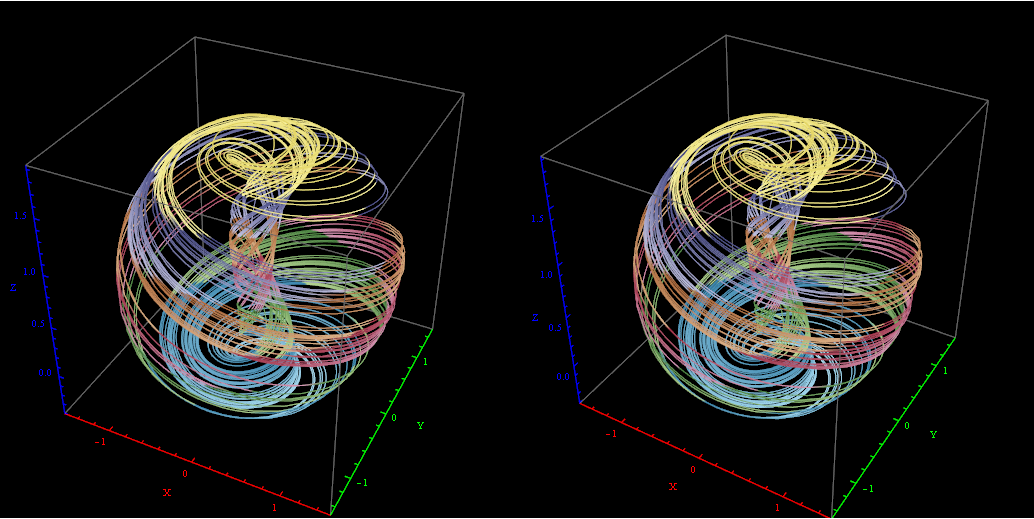
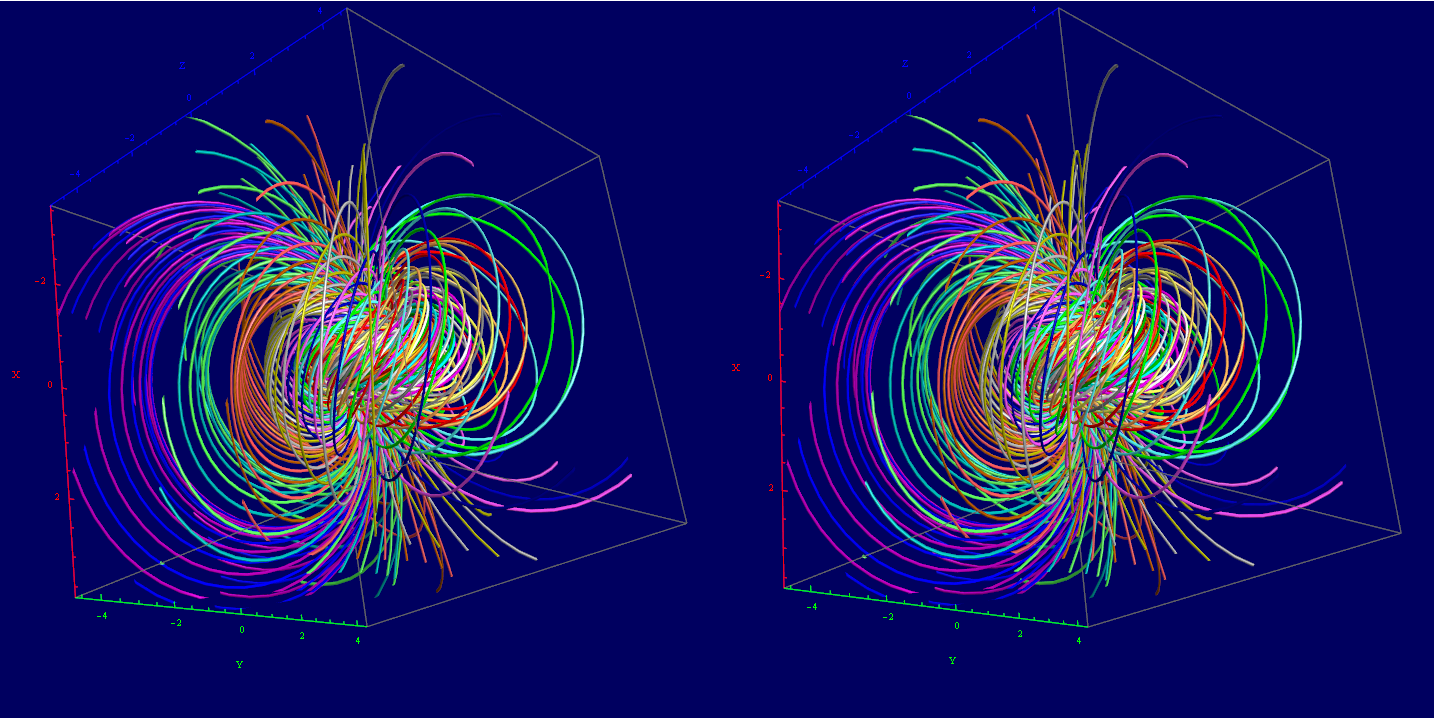
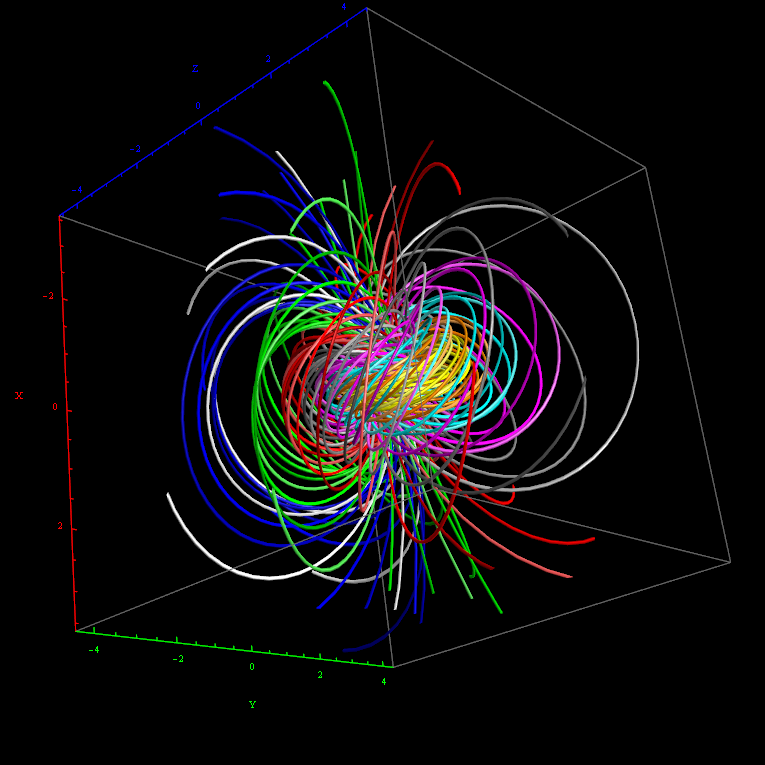
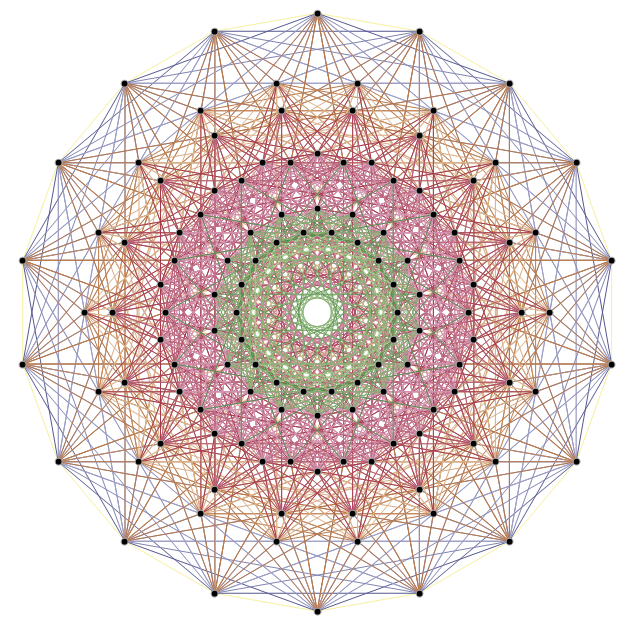
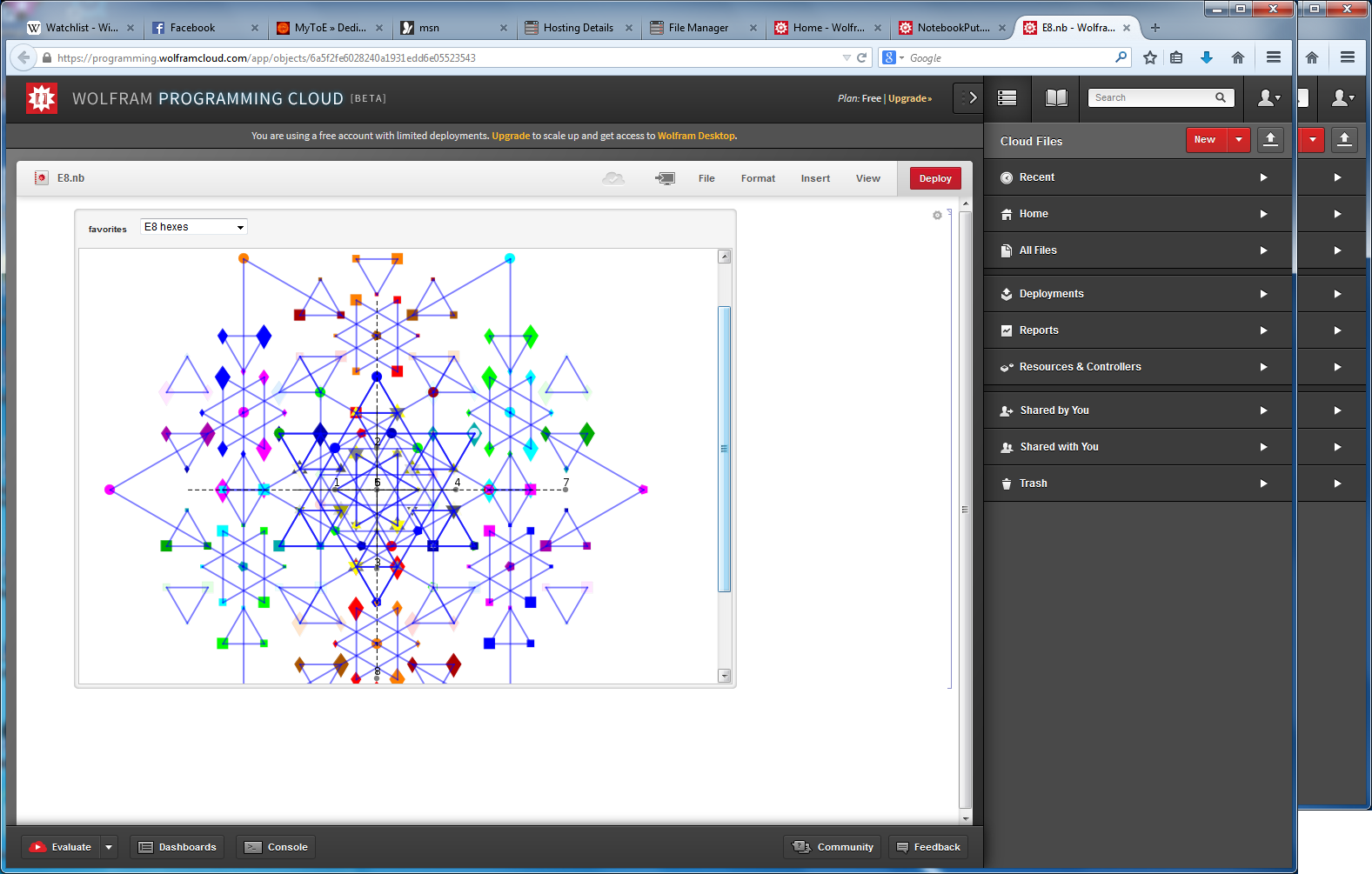
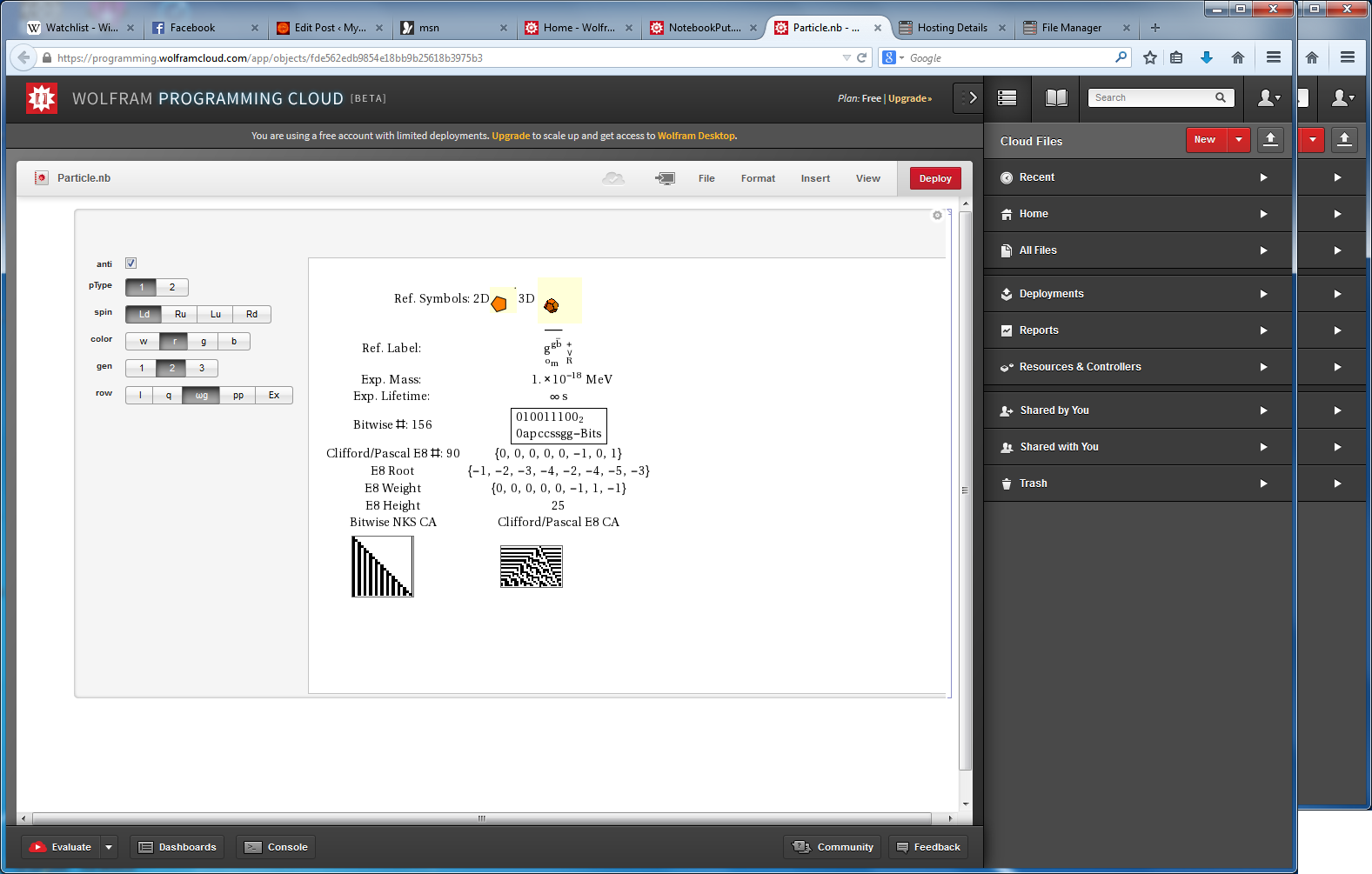
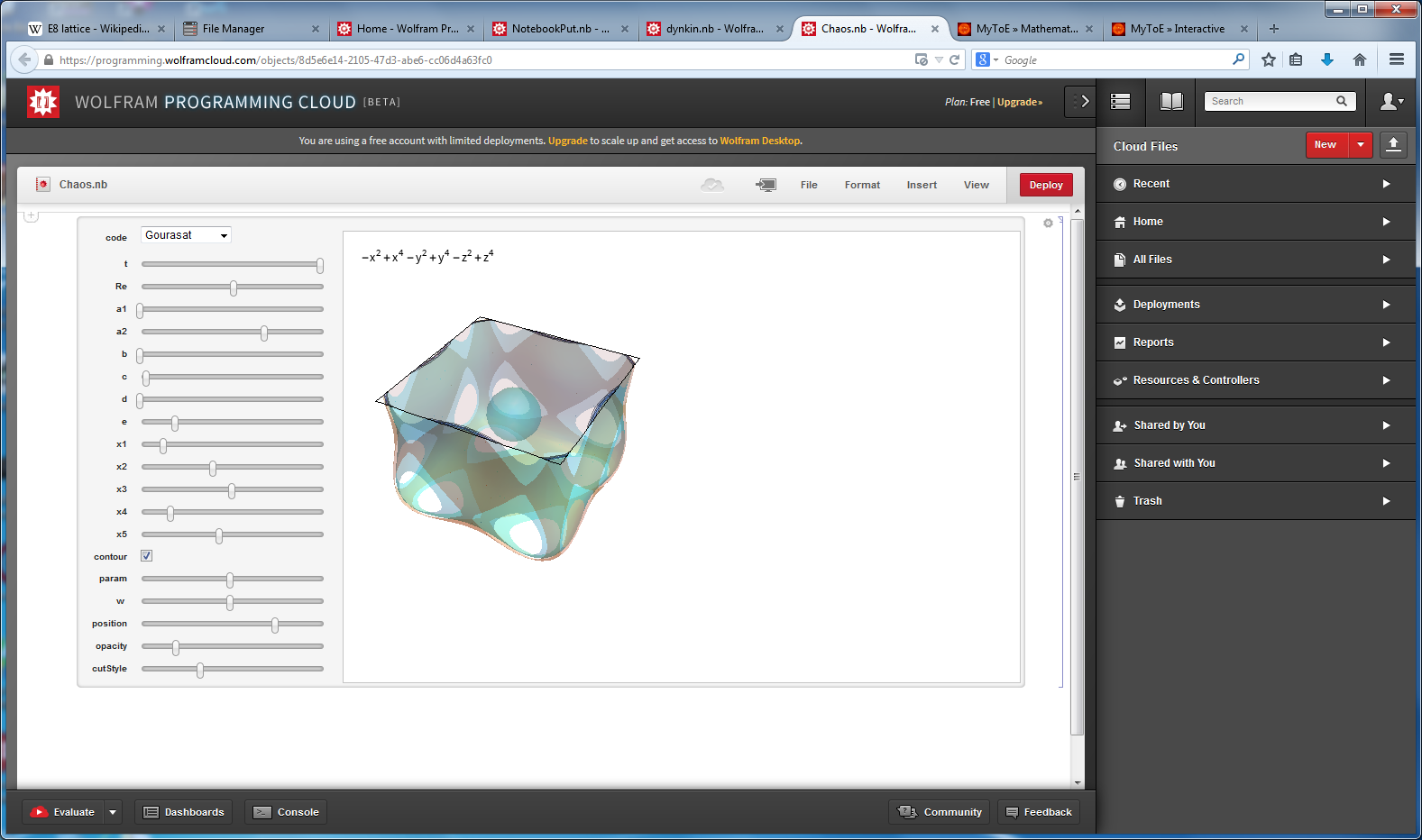
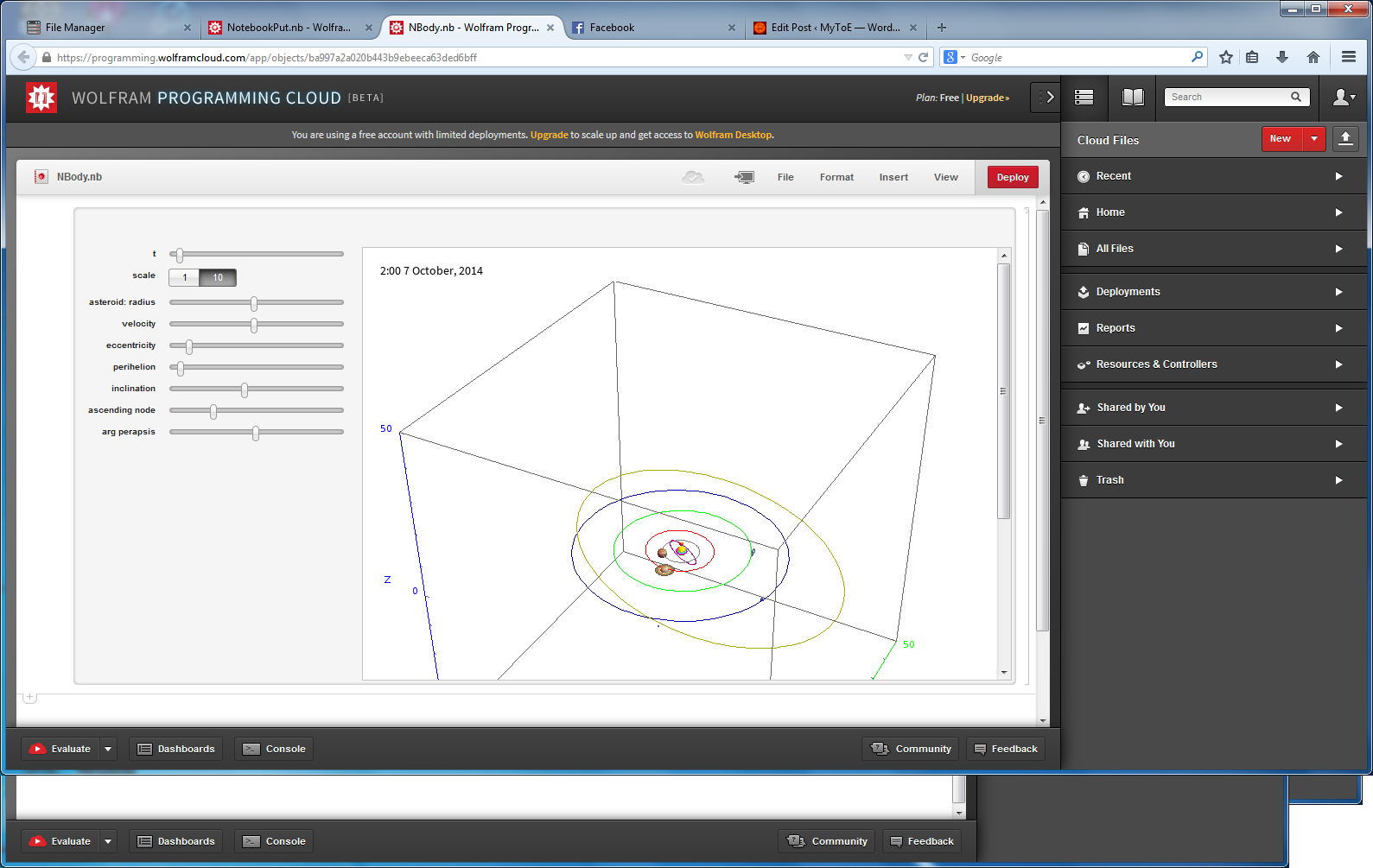
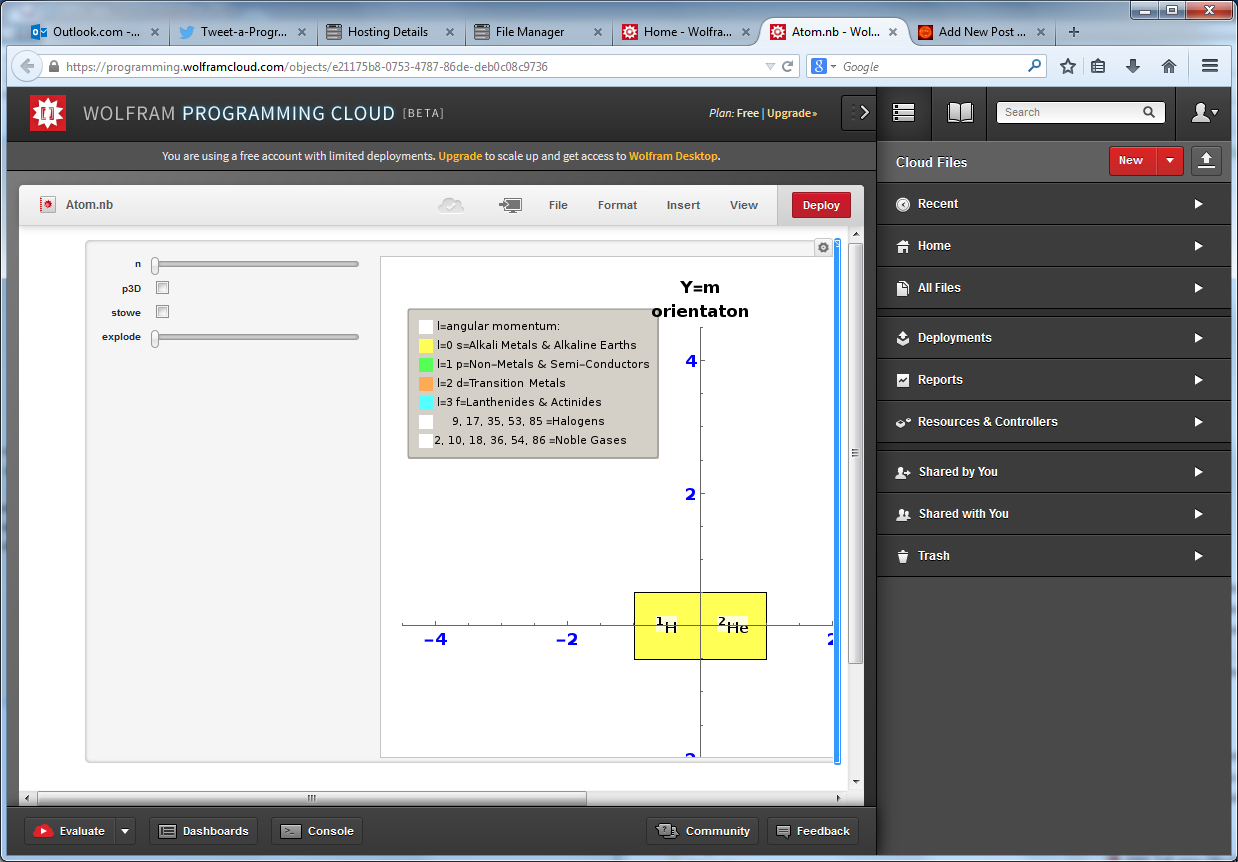
I’ve added some new features to my VisibLie_E8 ToE Demonstration. Some of it comes from Richard Hennigan’s Rotating The Hopf Fibration and Enrique Zeleny’s A Collection Of Chaotic Attractors . These are excellent demonstrations that I’ve now included with the features of my integrated ToE demonstration, since they are not only great visualizations, but relate to the high-dimensional physics of E8, octonions and their projections. This gives the opportunity to change the background and color schemes, as well as output 3D models or stereoscopic L/R and red-cyan anaglyph images.
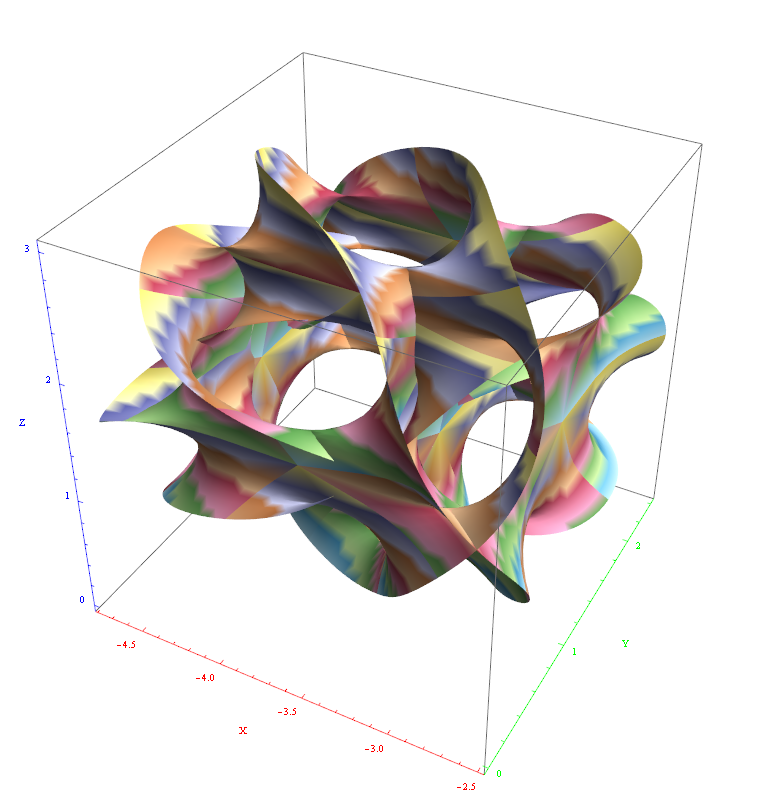
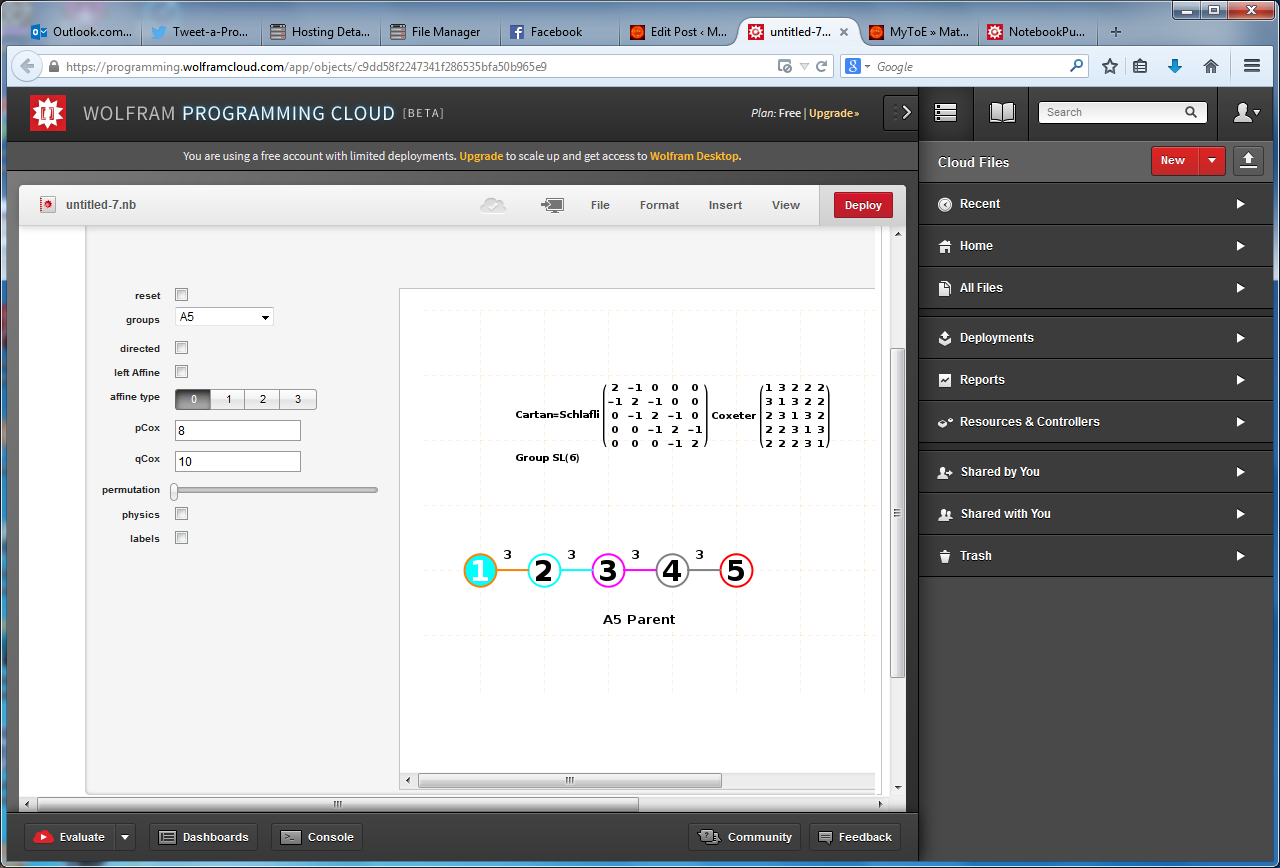
I’ve also used David Madore’s help to calculate the symbolic value of the E7 18-gon and 20-gon symmetries of E8. It uses the nth roots of unity (18 and 20, in this case) and applies a recursive dot product matrix based on the Weyl group centralizer elements of a given conjugacy class of E8. I ended up using a combination of Mathematica Group Theory built-in functions, SuperLie and also LieART packages. These symbolic projection values are:
Mathematica MyToE on the Wolfram Cloud
I am playing around with the Wolfram Tweet-a-Program, and the Wolfram Language (i.e. Mathematica) on the Wolfram Cloud.
What’s really cool is that you can now interact with advanced math and HPC on your phone/tablet.
Here are a few results…
@Wolframtap
BTW – you will need a WolframID (and be logged into WolframCloud.com) to interact with these pages.
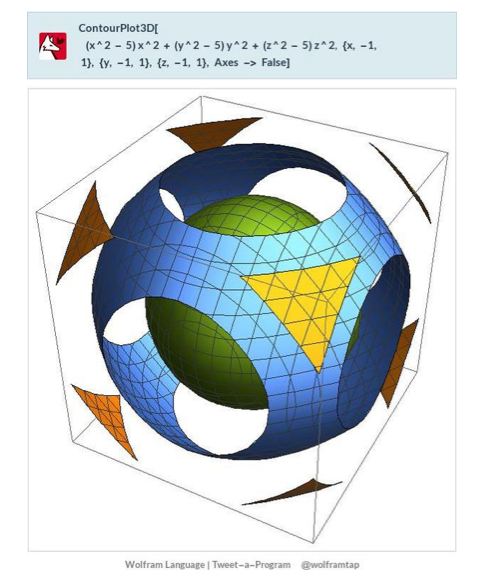
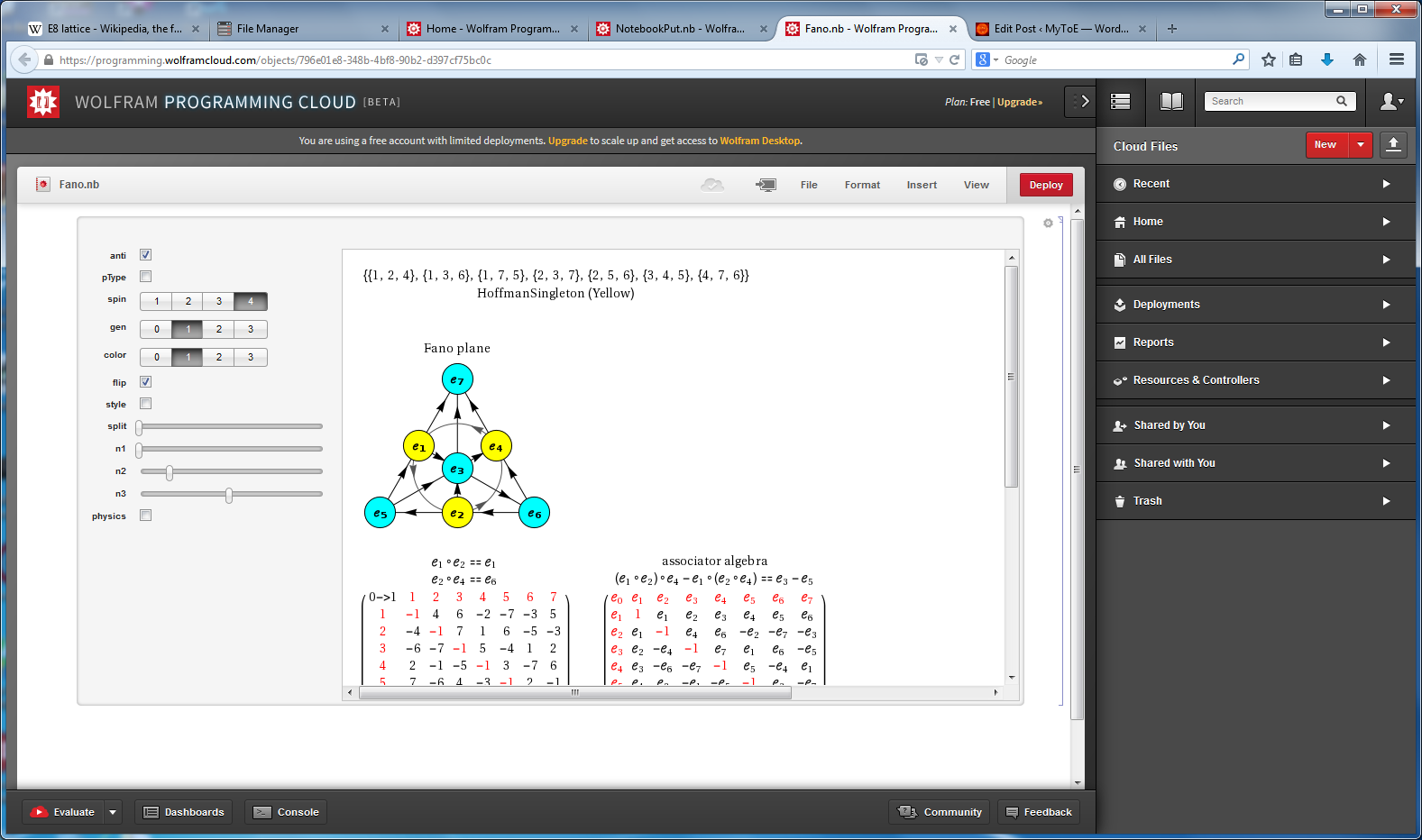
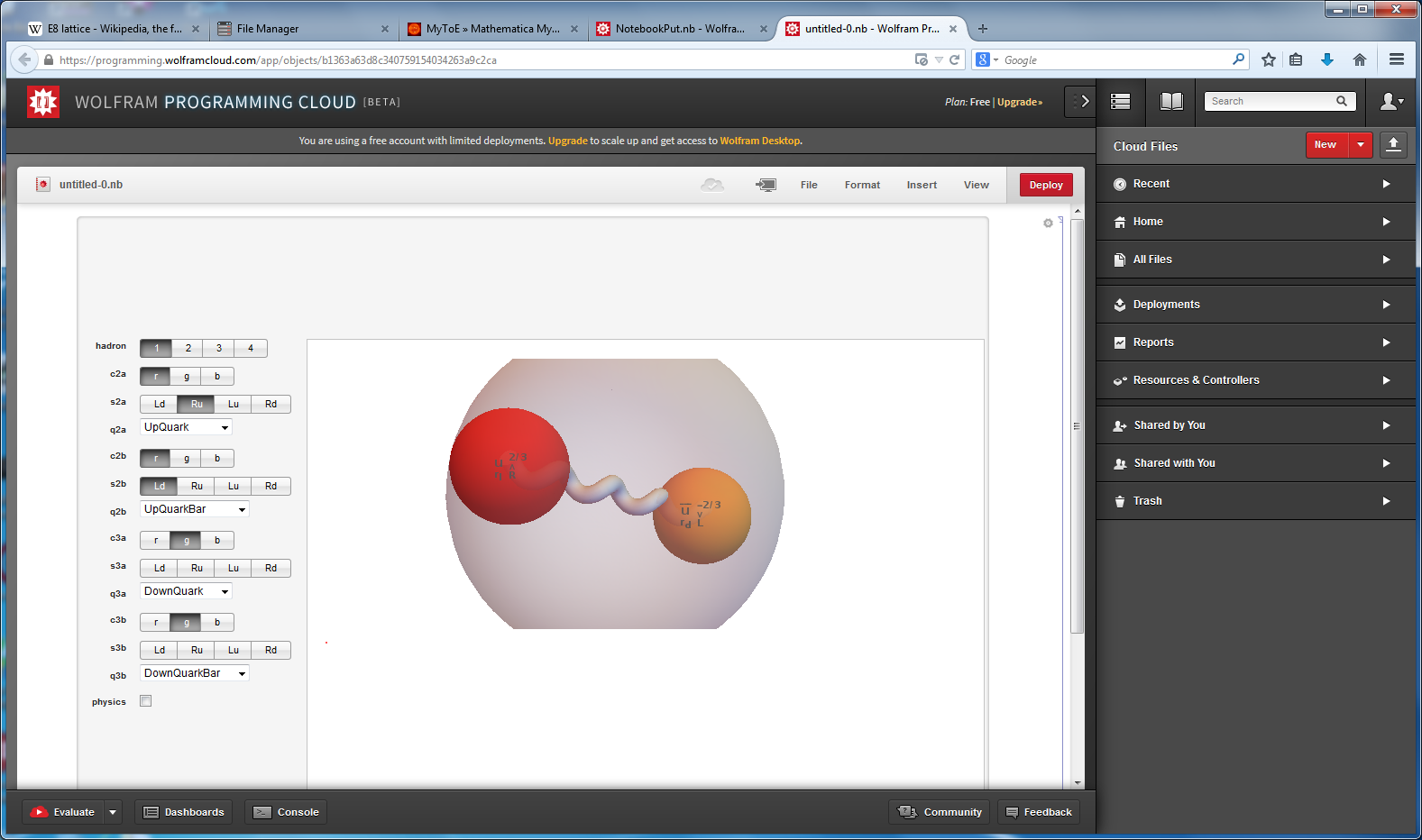
Octonions: The Fano Plane & Cubic
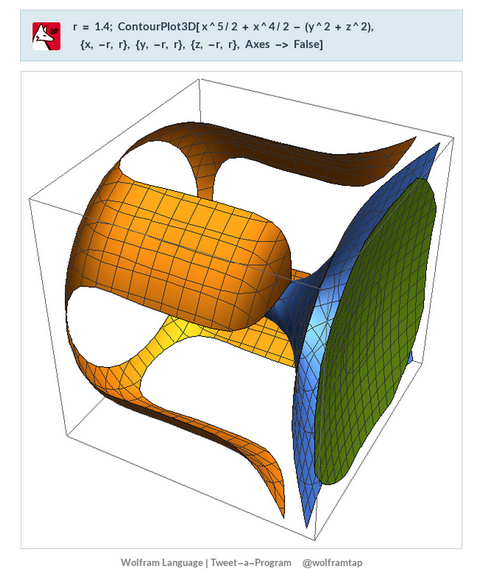
Navier-Stokes Chaos Theory, 6D Calabi-Yau and 3D/4D Surface visualizations
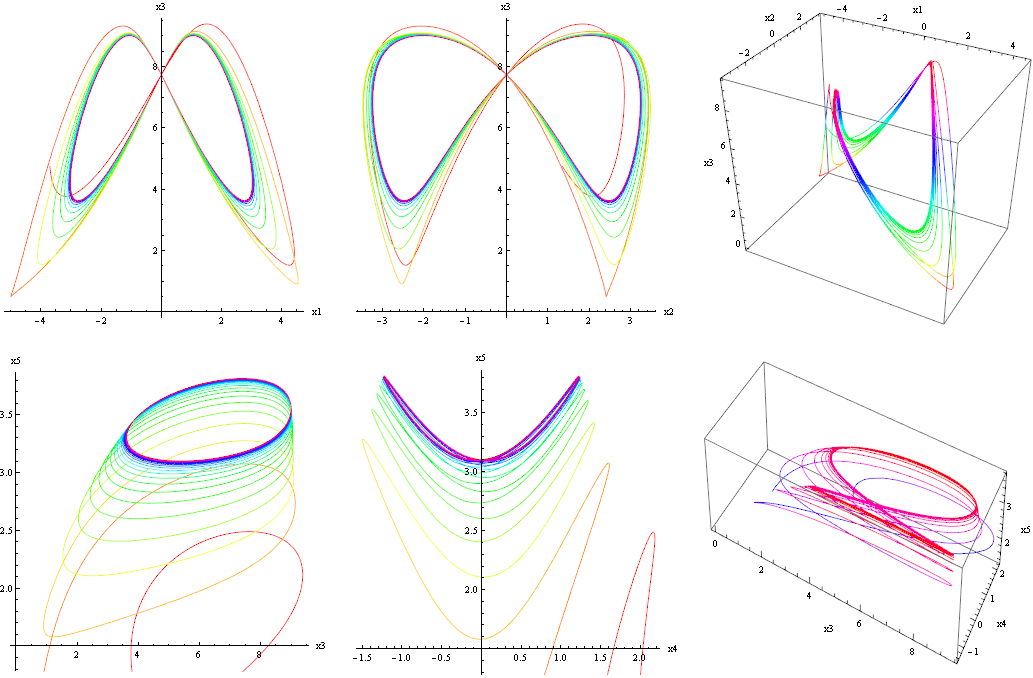
Another Wolfram Demonstration by Zeleny added – Navier-Stokes Chaos in 3D
I’ve added an interactive (Chaos) pane (#7). It is taken from Zeleny’s Five Mode Truncation Of The Navier-Stokes Equations. It now includes 2D and 3D visualizations of these equations. This will be a basis for study of a superfluid space-time theory to merge GR with QM.